Chapter 11
The Photoelectric Effect, the Compton Effect
and Blackbody Radiation
"Anyone
who conducts an argument by appealing to authority is not using his
intelligence;
he is just using his memory."
Leonardo
Da Vinci (1452-1519)
So Far
Up to
this point in the book there have several proofs of the ether drag theory.
But
this is not the end of the debate. There are still things to understand and
this
chapter
will deal with three experiments frequently used to prove the photon
theory,
or at least prove the particle nature of light (light obviously does have
particle
properties).
The Photoelectric Effect
Einstein
did not discover the photoelectric effect, but he did develop the formulas
and
published a paper on the photoelectric effect in 1905. Heinrich Rudolf Hertz
(1847
- 1894) had discovered the photoelectric effect in 1887. Millikan later
proved
that Einstein's formulas were correct. Both Einstein and Millikan won
Nobel
Prizes for their work on the photoelectric effect.
The
photoelectric effect involved the knocking of electrons off of the surface of
metal
plates in a vacuum. The classical model for ether predicted that the
amplitude
or intensity of light would be the determining factor
in how many
electrons
were knocked off of the surface of the metal plate. For example, with
ocean
waves the amplitude of the waves is what provides the bulk of the energy.
The frequency
of ocean waves is fairly irrelevant in providing large amounts of
energy.
This is logical because the amplitude or height of several large ocean
waves
delivers far more water, and thus more energy, than does a larger number
of
much smaller waves. Thus, if ether is a wave, then some people concluded
that
it should be the amplitude of the waves that provided the bulk of the energy,
not
the frequency.
While
it is common to use physical metaphors to prove or disprove a theory, one
must
be careful when using physical metaphors when dealing with ether,
because
light is an electromagnetic wave or signal, not the cumulative effect of
101
moving,
physical particles. Thus, light is not even necessarily like sound,
because
light is an electromagnetic "bumping," not a physical
"bumping."
What the
photoelectric effect proves is that it is the color or frequency of
light
that
provides the energy of light to knock electrons off of metal plates (for a
particular
type of metal), not the amplitude of the light. For example, for
some
metals
red light will not change the electron equilibrium of the metal plates, but
blue
light will. The amplitude of the light is of no importance, except that if the
frequency
of the lightwave is in the correct range, the amplitude of the light will
determine
how many electrons are released.
Using
the logic of the ocean example to prove the photon theory gives the
impression
that the photoelectric effect proves that light does not have
wave
properties.
This is absurd. It is well known that light has wave properties and
particle
properties. Furthermore, if it is the frequency of light that
causes
electrons
to get knocked off, then it is the wave properties of light that
causes
electrons
to get knocked off (the term "frequency" and "wave" mean
the same
thing).
The question is, is it the wave nature of ether or is it the wave nature of
photons
that knocks the electrons off?
What
is it about this experiment that can separate the two theories? Physicists
have a
difficult time explaining how particles can have wave properties (e.g.
Young's
dual-slit experiment, Poisson's spot, etc.), and suddenly it is the wave
properties
of photons that are knocking electrons off of metal surfaces, but the
wave
properties of ether cannot!
Furthermore,
if the amplitude of ether waves would be expected to knock the
electrons
off of the plates, then logically it would be the amplitude of photon
waves
that would also knock electrons off of the plates. Thus, if the ether theory
is
eliminated, then why shouldn't the photon theory also be eliminated? There is
clearly
a double standard in this debate, as there usually is.
But
understanding the photoelectric effect, relative to ether or photons, was not
fully
understood until 1927, when it was discovered that electrons have wave
properties.
In 1923 or 1924 (depending on what event you are talking about),
Louis
De Broglie speculated that matter has wave properties. But it was not until
1927
that it was accidentally discovered that electrons have wave properties. I
quote:
"The wave nature of the electron was experimentally confirmed in 1927 by
C.J.
Davisson, C.H. Kunsman and L.H. Germer in the United States and by G.P.
Thomson
(the son of J.J. Thomson) in Aberdeen, Scotland. De Broglie's theory of
electron
matter waves was later used by Schrödinger, Dirac and others to
develop
wave mechanics."
(http://www-gap.dcs.st-and.ac.uk/~history/Mathematicians/Broglie.html)
Thus
we are dealing with light, which has wave properties, and we are dealing
with electrons,
which also have wave properties. Thus, it is logical that in the
102
unique
case of the photoelectric effect the frequency of the light is more
important
than is the amplitude of light, whether the ether or photon theory is
correct.
In
other words, if electrons have wave properties, they also have frequency
properties.
Thus it makes perfect sense that the frequency of light is what can
provide
enough energy to release excessive amounts of electrons from an atom,
because
electrons have frequency properties also.
In
reality, electrons are constantly in motion and are constantly being released
from a
metal plate (in the experiment two metal plates are placed close to each
other
and they are attached via a wire and meter). Eventually equilibrium will be
reached
when an equal number of electrons are moving back and forth through
the
vacuum and through the wire. Bombarding one plate with a beam of light
with
the right frequency (depending on the metal) provides enough energy to
release
electrons and destroy the equilibrium.
Maxwell,
who was one of the foremost supporters of ether in the 19th century,
stated
emphatically that lightwaves were electromagnetic waves, not physical or
material
waves, such as the ocean. Thus, why should the scientific community
relate
the properties of a physical wave (e.g. the ocean) to the properties of an
electromagnetic
wave (i.e. light), especially when electrons are involved? That is
like
expecting a radio signal and an arrow to have the same properties!
Nevertheless,
there is a physical example of two frequencies joining together to
accomplish
some task. This is an example of a signal through air, sound, having
an
affect on a physical object. Light is a signal through ether, and it too can
have
an affect
on a physical object.
It is
well known that the pitch or frequency of sound can break certain types of
glass.
I quote from an internet site:
"First,
the type of glass matters. As Louis Bloomfield of How Things Work points
out,
the glass usually found in windowpanes and cups is relatively soft, so it
vibrates
poorly and has no strong natural frequencies. If you tap a glass of this
sort,
all you hear is a dull "thunk" sound. There's nothing with which a
highpitched
sound
can resonate. Crystal is better suited because it vibrates well and
emits
a clear tone when you tap it. Lead oxide is added to the glass, making the
resulting
crystal stronger than ordinary glass. Crystal wine glasses work well for
this
experiment because, in addition to being crystal, they are thin and delicate,
and
the tubular shape enhances the sound frequency. The real trick to breaking
glass
with sound is to match the sound's frequency to that of the glass. You
might be
able to do this with a scream, but it's easier for a singer with perfect
pitch
to create the right note, especially if that singer's voice is amplified. Each
glass
will have a slightly different natural frequency due to minute variations in
shape
and composition. When the high-pitched sound and the glass resonate, it
103
causes
the glass to vibrate. If the singer keeps singing the same note at high
volume,
eventually the glass will vibrate itself into pieces."
(http://ask.yahoo.com/ask/20011212.html)
Thus,
knowing that ether has frequency properties (as sound does), and knowing
the
electrons have frequency properties (as crystal does), we can see from the
physical
world that there should be no surprise that the ether theory can easily
explain
this phenomenon.
But
let us also look at this phenomenon from a different perspective. If we
assume
that ether exists, then when an electron drops from one quantum level to
a
lower level, we know that this motion can "bump" or stimulate the
ethons
surrounding
the atom at a specific frequency. In other words, the changing of
quantum
levels generates a specific frequency wave of light.
Therefore
it stands to reason that the reverse is also true. The frequency of light
can
cause electrons to change quantum levels. In fact, we know that it is true
because
atoms absorb energy from ethons under the right conditions. Since an
electron
is freed from an atom when it jumps to a quantum level that doesn't exist
for
that type of atom, it makes perfect sense that the frequency of light is what
dislodges
electrons from atoms.
(Note:
In stating the above paragraphs, it should be emphasized that it is not
known
whether the electron drop "bumps" the ether or whether it is the
energy in
the
ether that causes the electron to drop down. In terms of bumping the
electron
up, it is most certainly the ether that initiates this event.)
In
reality, the discovery that electrons have wave properties is just one of the
many
discoveries that should have reopened the ether-photon debate, but of
course
it did not.
The Compton Effect
The
Compton effect is a little more complicated. It involves the scattering of
electrons
and the resultant wavelengths associated with the angles beyond the
collision.
I
quote from the well-known book on light by Ditchburn:
"Compton's
original experiments deal with average effects due to large
numbers
of collisions. They cannot, therefore, give direct
evidence concerning
the
change of momentum in a single collision. ... It is possible to
obtain the
change
of wavelength from a purely wave-theory [ether] by assuming that the
scattering
is a double process in which the light is absorbed and is then emitted
104
by the
moving electron. The change of wavelength is then ascribed to the
Doppler
effect." [italics, underline added][29]
In
fact, whether the photon or ether theory is correct, the electrons jump off of
the
metal
because of intense localized energy (e.g. heat). What generates this
intense
energy can be explained by either the photon or ether theory.
To
better understand how, let us consider an example from the sports world.
The Pool Table Example
Image
a pool table that is one hundred feet long and five feet wide. Suppose
there
is a straight line of pool balls, each touching the other, on one side of the
table
on the long axis. This line of pool balls goes from within one foot of the
near
side of the pool table to within one foot of the far side of the pool table. In
other
words, the line of pool balls is ninety-eight feet long.
Now
image that there are two cue balls on the near end of the table, three inches
from
the near cushion. One of the cue balls is lined up with the long line of pool
balls
and the other one has no pool balls between it and the far cushion, almost a
hundred
feet away. Let us assume that the last of the pool balls in the long line
of
pool balls is also a cue ball.
Let us
assume the cue ball in the long line of pool balls is hit by a man, and the
other
cue ball is hit by a woman. The cue ball that is hit by the male pool player
only goes
a few inches, but the last of the pool balls in the long line, which is also
a cue
ball, hits the far cushion.
Now
suppose there is a curtain at the far end of the pool table that is six inches
from
the far cushion, such that no part of the pool table can be seen (by
someone
behind the curtain) except for the last six inches of the pool table.
See
the graphic on the next page:
105
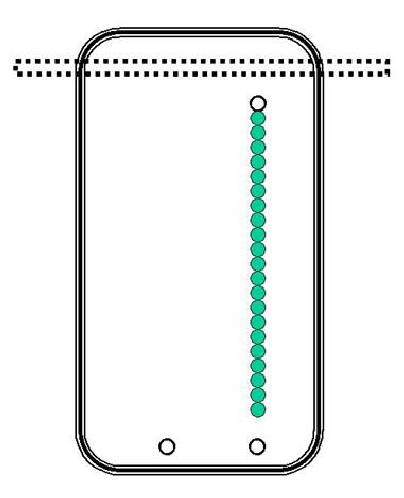
106
Given
the same amount of energy from the two pool players, the long line of pool
balls
will cause a ball to hit the far cushion much more quickly than the cue ball
that
has to travel the entire distance. And with more energy, given the same hit
of the
two pool players.
Now
let us suppose there is a judge at the far end of the pool table who can only
see
the last six inches of the pool table because of the curtain. Now suppose
that
the two pool players each hits their cue ball at such an energy level and with
such
timing, that both the woman's cue ball and the last of the pool balls in the
long
line, which is also a cue ball, hit the far cushion at exactly the same time
and
with
the same energy level (i.e. the same velocity in this case).
How
can the judge behind the curtain tell which cue ball was hit by the man (i.e.
the
cue ball that resulted indirectly from the man's hit) or was hit by the woman?
The
judge cannot tell.
The
long line of pool balls (i.e. the "wave") obviously represents the
ether theory
of
light. The single cue ball (i.e. the "particle") hit by the woman
represents the
photon
theory of light.
This
simple example contains a very profound message: since the long line of
pool
balls (the "wave") is composed exclusively of
"particles" (i.e. pool balls),
the
long line of pool balls has "particle" properties identical to the
"particle"
properties
of the cue ball hit by the woman! In fact it is impossible to tell which
pool
ball is the "wave" cue ball (from the long line of pool balls) or
which is the
"particle"
cue ball (the cue ball that travels the entire distance).
Of
course, the actual "bumping" of contiguous ethons is exclusively
electromagnetic,
not physical, thus the many properties of pool balls and ethons,
such
as the dispersion properties of pool balls versus light, would not necessarily
be the
same.
But
there is another serious problem with the Compton Effect:
Problems With Determining How Light Travels
If an
equal amount of energy is observed resulting from the two pool players (at
the
far cushion), it is impossible for the judge to distinguish between which
energy
level resulted from the "wave" of pool balls and which resulted from
the
"particle"
cue ball. But what if the judge could observe the energy applied by
both
the man and the woman, and the judge could observe how much energy is
applied
to the far wall?
107
For
example, if both the man and the woman hit their respective balls with an
equal
amount of energy, then the amount of energy at the far wall will be stronger
for
the long line of pool balls. Thus, if the judge knows that both cue balls are
hit
with
the same energy level, and if the judge understands that one cue ball travels
the
entire distance by itself, and the other cue ball hits a long line of pool
balls,
then
the judge, based on his own experiments, can tell which side of the table the
long
line of pool balls is on!
Likewise,
if the judge knows that both energy levels at the far cushion are equal,
but
the judge knows how much energy, and when, each pool player hit their
respective
cue ball, then the judge can tell which side of the table has the long
line
of pool balls.
In
short, because the long line of pool balls is more efficient than the single
cue
ball,
that has to travel the entire distance by itself, then knowing how much
energy
is exerted and how much energy is measured at the end of the table will
tell the
judge which side of the table has the long line of pool balls.
With
this in mind, it should be easy to determine whether light travels by photons
or
ether. Unfortunately, it is not as easy as it sounds.
With
the pool table example, we can do simple experiments to determine, under
specific
conditions, just how efficient the long line of pool balls is, compared to
the
woman’s efforts. We don’t have that luxury with light because we cannot
calculate
how efficient ether would be compared to photons because:
1) If
ether exists, we cannot create photons for our experiments, nor can we
create
an "ether vacuum" for our photons to travel through, or
2) If
photons exist, we cannot create ether for our experiments.
In
other words, we cannot compare them both side-by-side because both of them
do not
exist. To elaborate, whether light travels by photons or a chain reaction
inside
of ether, the energy that hits an atom (such as in the Compton Effect) is an
"electromagnetic"
energy, not a physical or mechanical energy. It is also a wave,
since
photons are claimed to have wave properties also. How is it possible to
theoretically
calculate the efficiency of an electromagnetic jolt from a free flying
particle
(i.e. a photon) versus an electromagnetic jolt from a chain reaction of
charges
that travel in the ether? We only have a single final number, but that
single
final number does not tell us anything because we have no number to
compare
it to.
As
with the photoelectric, there is really nothing about the Compton Effect,
except
assumptions, that helps us determine whether light is a particle or a
signal.
108
Blackbody Radiation
In the
photon theory of light, each photon is a small “light quanta,” or particle of
light.
Thus each photon is discrete in nature. One of the experiments that was
felt
to demonstrate the discrete nature of light energy was the "blackbody
radiation"
or "cavity radiation" experiment.
The
blackbody radiation experiment consists of a metal box, completely
enclosed,
but with a small window on it so someone can see inside. The box is
slowly
heated up and the observer can see the colors in the box change as the
box
heats up. The term "blackbody" refers to the color of the inside of
the metal
box,
the term "radiation" refers to the light that is emitted as the box
heats up.
The
phenomenon of blackbody radiation was not discovered by Max Planck, in
fact
he was not the first person to work on the formulas for blackbody radiation.
Many
scientists studied the blackbody radiation experiments, but no one was
able
to come up with a formula that would accurately predict the entire frequency
distribution
of the blackbody spectrum for any temperature.
The
first to have some success at predicting this distribution were John Rayleigh
and
James Jeans. The Rayleigh-Jeans formula was based on classical theory.
The
formula worked well for the lower frequencies, but since they used an
exponential
function, the function did not work well at the higher frequencies.
This
is because a typical blackbody radiation distribution curve looks like a
distorted
"bell" curve, thus at the higher frequencies, instead of an
exponential
rise,
the curve drops.
Their
formulas were vastly improved by Wilhelm Weir, whose formulas worked
well
at the higher frequencies, but were not exactly correct at the lower
frequencies.
Max Planck made a very minor, but profound, change to the Weir
formula
and was successful at matching the entire frequency distribution.[22-Chapter
4]
But a
formula does not explain "why" something happens the way it does.
Upon
a
great deal of further analysis, Planck concluded that the formula worked
because
light had quantum or discrete energy levels (i.e. he concluded that light
frequencies
are continuous, but their energy levels are
discrete). His discovery
was
not well received at first. But Einstein took him seriously and came up with
the
"little box" thought experiment to justify both his own belief in
photons
(according
to some researchers Einstein had disavowed the ether theory in the
1890s)
and the discrete nature of light that Planck had discovered.
Einstein
pictured in his mind a "little box" that had a small hole in one
side, that
was
placed inside of Planck's big blackbody radiation box. Einstein imagined
that
individual light particles randomly went into and out of this "little
box," thus
109
creating
discrete energy levels within the little box. In other words, if
there were
only a
handful of photons in the box, the movement of a single photon into or out
of the
little box would cause discrete changes in the energy level inside the box (I
am
simplifying his arguments).
As a
purely academic matter, Einstein's "little box" would have to be much
smaller
than a single hydrogen atom, and perhaps smaller than a single electron,
to
contain only a few photons. If the "little box" was one cubic
centimeter (and
the
hole was proportionally sized), many, many photons would be going into and
out of
the little box at any given time. In this case the movement of a single
photon
would not have been detectable by Planck.
In
other words, Planck's crude equipment could not detect the movement of a
single
photon; it could only detect the average, simultaneous motion of many,
many
trillions of photons. And even then, it was the formula, not any observed
phenomenon
by Planck, that pointed towards discrete energy levels. In other
words,
the discrete nature of photons could not have been the cause of the
discrete
energy in the experiment because there were far too many photons
(assuming
the photon theory) for his equipment to detect the result of the motion
of
individual photons.
To
understand more about discrete energy levels, let us continue the pool table
example.
Let us suppose there is a machine that can hit cue balls. Suppose this
machine
has a finite number of settings, say 100 different energy settings. In
other
words, the machine can only hit a cue ball with one of 100 different energy
levels.
Let us
put this machine behind the cue ball that is lined up with the long line of
pool
balls. Here is the question: "how many different energy levels will be
observed
by the judge at the far end of the table if we use the machine to hit the
cue
ball lined up with the long line of pool balls?" If the machine has 100
settings,
the judge will only observe 100 different energy levels of arriving cue
balls,
even if we do the experiment a million times.
If
atoms only generate a finite number of energy levels, meaning if there are only
a
finite number of possible electron drops from high quantum levels to lower
quantum
levels, then why would anyone expect that if light travels via ether that
light
would have a continuous number of different energy levels? It is interesting
that
light has continuous wavelengths, but there are only a finite number of
possible
quantum drops.
There
are three items that control whether a type of energy is perceived to be
continuous
or discrete:
110
The
1)
"energy source" generates the energy (i.e. the electrons
in atoms) and
transfers
the energy to an ...
2)
"energy carrier," which carries and transfers the
energy (i.e. the photon or
ether)
until an ...
3)
"energy detector or absorber" calculates or
absorbs the arriving energy
level
(this would be a spectroscope in the case of Planck's blackbody radiation
experiment).
If all
three of these items can handle continuous energy, then the energy detector
may observe
continuous energy levels (i.e. the formula that describes the
resulting
distributions may be consistent with continuous functions). If any one of
these
items can only handle discrete or finite levels of energy, then only discrete
energy
levels, meaning a finite number of energy levels, will result.
Einstein
focused his attention on the "energy carrier," however; it is
currently
accepted
that the "energy source," namely atoms, can only provide discrete
energy
levels. Thus, even if a photon or an ethon could carry a continuous
number
of energy levels (and why couldn’t they?), only a discrete or finite
number
of energy levels would be observed whether light travels by photons or
ether.
It is
absolutely incredible that blackbody radiation was used as a "proof"
of the
photon
theory when Bohr's model for quantum electron levels (1913) was
developed
long before the photon theory was accepted in 1924. At the time Bohr
came
up with his first model, the ether theory was still well entrenched in the
scientific
world. Thus it should have surprised no one that the "energy source"
was a
discrete energy source, and thus it should be expected that discrete
energy
levels would be observed. How can a discrete energy level be converted
into a
continuum? It can't unless there are averages of many, many individual
events.
No
matter whether light is photons or ether, what goes on inside of the blackbody
box
involves many, many photons or many, many signals, thus how does
blackbody
radiation prove anything about light?
Is it
possible a particle can "carry" a continuous number of energy levels?
We
just
saw that with the pool table example. If a human hits one of the cue balls, a
continuous
number of energy levels will be transferred by either of the cue balls.
On the
other hand, if a machine with only a finite number of energy levels hits
either
of the cue balls, then only a discrete number of different energy levels will
be
transferred by either cue ball.
Let us
look at another wave example. Let us consider a guitar being played by a
robot.
Suppose the robot has the ability to pinch or press the guitar strings
111
anywhere
on the "neck" of the guitar (i.e. at a continuous number of points).
But
suppose
the robot can only pluck the strings with any one of 20 different energy
levels.
In other words, the robot can play a continuous number of different
frequencies
but only with a finite number of different energy levels.
We now
have these items to consider (in parenthesis is a note whether this item
can
handle a "discrete" or "continuous" number of frequencies
and energy
levels):
1) The
robots "fingers" (discrete energy levels, continuous frequencies),
2) The
guitar strings, which is the "energy source" (continuous wave for
both, if
played
by a human),
3) The
air, which is the "energy carrier" for sound (continuous wave for
both), and
4) Our
ears, which is the "energy detector" (continuous wave for both).
Every
item in the list can handle a continuous number of different frequencies
and
energy levels, except for item number one.
But
note in this case that it is not the "energy source" (i.e. the
strings), nor the
"energy
carrier," (i.e. the air), nor the "energy detector” (i.e. our ears)
that causes
the
discrete number of different energy levels! All three of these things can
handle
continuous energy levels and continuous frequencies. It is something
related
to the experiment (i.e. the robot) that causes the discrete energy levels.
Thus
we see that there is a fourth thing that can cause discrete energy levels -
the
physical facilities and environment of the experiment.
Suppose,
for example, that atoms (the "energy source") can create continuous
energy
levels (which they apparently can't) and that photons or ethons can carry
continuous
energy levels and that the spectroscope can handle continuous
energy
levels. Is there a possibility that some physical aspect of the blackbody
experiment
itself caused the discrete energy levels?
With
all of this in mind, I quote from an email I received from Dr. Howard C.
Hayden,
Emeritus Professor of Physics, University of Connecticut, and former
Editor
of Galilean Electrodynamics:
"Thermodynamicists
looked at the blackbody curve (the data) and noticed the
similarity
to speed distributions in a gas. They tried to rig up a similar model to
explain
the blackbody curve. Nothing worked. One model fit the data at the red
end of
the spectrum but would end up with the ultraviolet catastrophe (infinite
power
at that end of the spectrum). Another model fit the blue end but failed at
the
red end. Along came Planck. Textbooks say he invoked E=nhf, but I don't
think
that's what he was thinking. It is more reasonable, to me at least, that he
regarded
the cavity as a resonant cavity, in which the diameter would be one
wavelength,
two wavelengths, etc., corresponding to f, 2f, 3f, etc. The BASIC
physics
he was using was resonance, and the DERIVED physics was E=nhf,
where
the f is merely the lowest frequency; more generally it would be E=hf.
When
Einstein proposed his solution for the photoelectric effect, Planck objected
112
strenuously.
The objection is obvious. Planck never meant for the E-M field to
be
quantized outside the cavity. Bohr seized upon Einstein's idea as a way to
explain
the hydrogen atom. So, in the picture you paint, the metal in the
photoelectric
effect is a quantum system, and the hydrogen atom is also.
However,
there is no reason for the E-M field itself to be quantized. At the very
least,
the experiments do not prove that it is."
113